How Politicians Try To Act Like Mathematicians
So these days you’ve probably been hearing an awesome bunch of government numbers thrown around in the news and debates – all concerning taxes and the federal budget and deficit spending and what not.
I certainly have, and while watching the last debate it put me in mind of how real mathematicians treat numbers, which I will share with you – and also a cool party ice breaker you may want to use sometime. So we’ll be mixing a bit of business with pleasure in this post.
Now hang in there with me because believe it or not – and you’ll be doubting it – I’m going to pull this all together in the end. And, as I said, will be giving you a line that will make you sound really smart at your next party.
So, pleasure first, I say — let’s get straight to the party ice breaker.
Imagine you’re at a cocktail party and there’s a lull in the conversation. You could be real cool and casually say something like, “Do you know that mathematicians often use imaginary numbers. They call one of them the number i. It represents the square root of negative one, which is impossible to exist.”
I’m sure you can see the possibilities here – and may even be surprised you haven’t thought of this clever line yourself. Because people are going to think you are really, really smart. Some may offer to go get you another drink – maybe all of them, all at once … ummm, bolting off rather quickly.
That aside, perhaps you’re wondering why watching the political debates caused me to think of mathematicians. Well, it was a natural train of thought, really, what with all the numbers being thrown around.
That is to say, mathematicians use numbers in their work (I hope you were sitting down for that). And they like numbers so much they actually give special names to different groups of numbers. Like, for example, the “counting” numbers. Yep, the ones you and I know so well, like 1, 2, 3, etc.
And what mathematicians think is so cool about the counting numbers is that if you add a counting number to another counting number you get … drum roll … another counting number, i.e. 2 + 2 = 4 (4 also being a counting number).
But then, being crazy mathematicians, they push the idea a bit and subtract some counting numbers and everything goes along just swimmingly until they accidentally subtract a bigger counting number from a smaller counting number — like if we tried to subtract 4 from 2.
Oops (as one politician recently said). There is no counting number for that answer. Rather poetically, I think, the above quoted politician was having trouble counting at that very moment.
So mathematicians just make up the number -2, or as they call these things, negative numbers (for our advanced readers, part of the “integer” number set). Mind you, no one in human history has ever seen a negative number, i.e. even cavemen could see 2 rocks, but absolutely no one has ever seen -2 rocks.
But no matter, the mathematicians just use negative numbers like they’re a real thing and press on. You’d think they’d quit while they’re ahead, but no, undaunted, they charge forward and then run into yet another problem when they try to divide a smaller number by a bigger one, like 3 divided by 4. So they just make up another set of numbers called “rational” numbers – hmmm, one could question their naming conventions I suppose.
And then one of them really pressed his luck and tried to do some square root thing with -1, meaning what number can we multiply by itself to get -1, and well, there just ain’t no number that will do that.
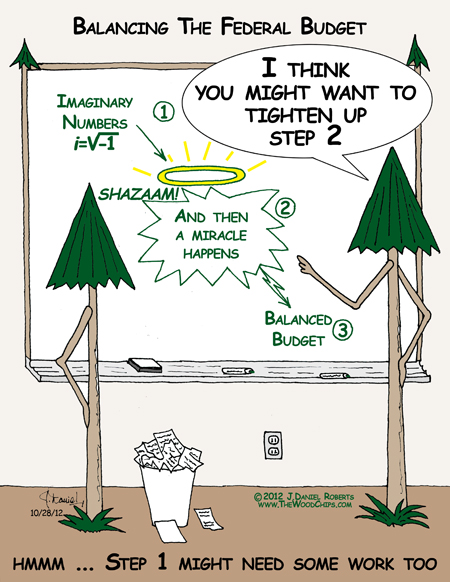
But no worries, the mathematicians just call this an imaginary number. Often they call it i and it looks like this …
i=√-1
So mathematicians are cool like this. When they bump into something indefinable, they just make stuff up.
Now this may sound a lot like the politicians in Washington these days, and I’ll admit there are great similarities. Indeed, you can see how all this readily popped into my head the other night while watching the political debates.
But there is a fine distinction. And that is to say that our politicians are up against something quite definable, i.e. don’t spend more than your income. That’s pretty definable, really.
And they still make stuff up.
Even a past president, with an Arkansas drawl, recently chided them on this fact by saying, “It’s just arithmetic.”
For example, here’s some plain ol’ arithmetic for you — the 2012 budget.
| Government Income |
$2,469,000,000,000 |
| Less: Government Spending |
3,796,000,000,000 |
| Government Deficit (oops) |
-$1,327,000,000,000 |
Hmmm … there’s one of those pesky negative numbers again … which comes from subtracting a bigger counting number from a smaller one, remember? I don’t think we’re in counting numbers anymore, Dorothy.
And yet somehow they all seem to propose some vague process of not raising taxes, or not reducing spending on programs, or neither, that, miracle of miracles, will result in a balanced budget with no more of those negative numbers. Sounds like imaginary numbers to me.
So anyhow, I came away from the whole thing a bit dazed and confused. But in a moment of clarity I came up with three guiding conclusions, which I call the Three Laws of Imaginary Numbers. You might want to print these out and tack them on your wall – or stick them to the refrigerator with those little magnet things. Here they are …
- The numbers you hear from most politicians are imaginary.
- When mathematicians use imaginary numbers its okay.
- When politicians use imaginary numbers its not okay.
Also, and supporting conclusion number 2, isn’t it fortunate that those crazy mathematicians invented negative numbers, because how else could we keep track of the national budget deficit. Now there’s a negative number with some real chops.
So there you have it and I think that pretty well sums up everything in the political and mathematical realm for the moment. I told you I’d pull it all together. So my work here is done, and I’ll be signing off for now.
But before I go, don’t forget my cool party tip about imaginary numbers – guaranteed to be a real ice breaker. And remember, you heard the Three Laws of Imaginary Numbers here first.
Pondering the imponderable, and confused on a higher plane – J. Daniel
P.S. I think it rather uncreative of mathematicians to call i an imaginary number. Why not something really cool – like m, for malarkey. As Russell Crowe, portraying Nobel Laureate mathematician John Nash, said in the movie A Beautiful Mind, “What’s the point of being crazy if you can’t have fun with it?”
P.P.S. Mathematicians kept pushing on this concept of number sets and eventually had to create the “irrational” number set as well. You may well understand how this fits with today’s thoughts and government in general, but it would have made for a really, really long article so I stopped at the imaginary numbers.
P.P.P.S. I’m not making this stuff up.
P.P.P.P.S. Source for budget numbers in article … http://en.wikipedia.org/wiki/2012_United_States_federal_budget.